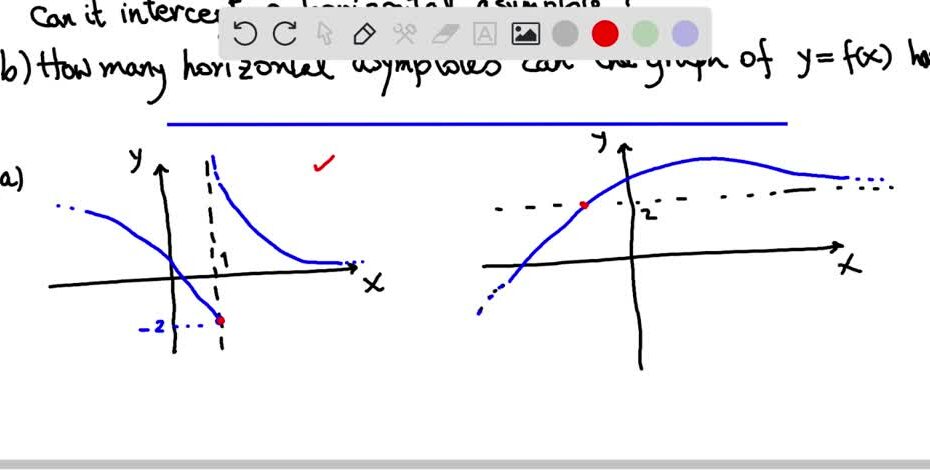
Can A Function Cross A Horizontal Asymptote? Exploring Limits In Mathematics

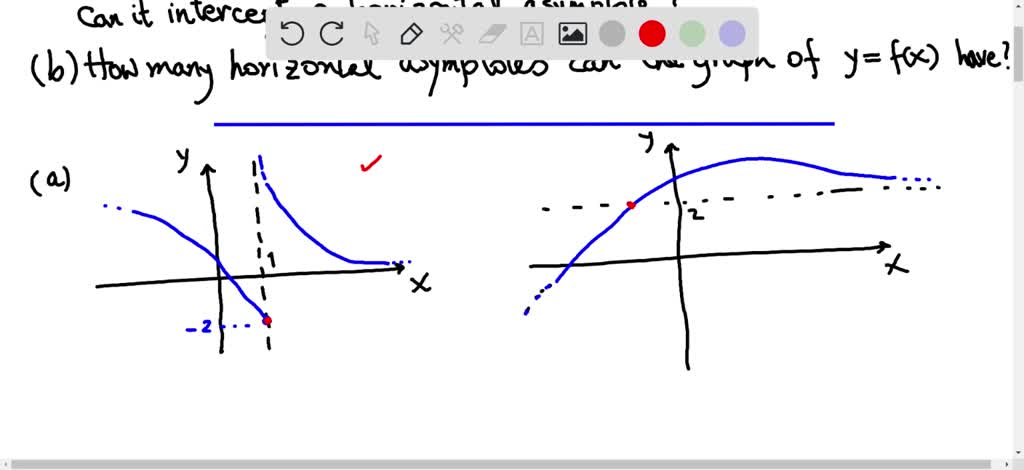
Can functions cross horizontal asymptotes? Horizontal asymptotes represent the behavior of a function as x-values approach positive or negative infinity. It is important to note that a rational function cannot cross a vertical asymptote because doing so would involve dividing by zero, which is mathematically undefined. However, when it comes to horizontal asymptotes, they serve as a guideline for the long-term behavior of the function as x-values become extremely large in either the positive or negative direction. Unlike vertical asymptotes, horizontal asymptotes can indeed be crossed by a function. This means that a function can approach and then cross a horizontal asymptote as it continues to evolve with increasing or decreasing x-values. This phenomenon adds an interesting dimension to understanding how functions behave in the long run.

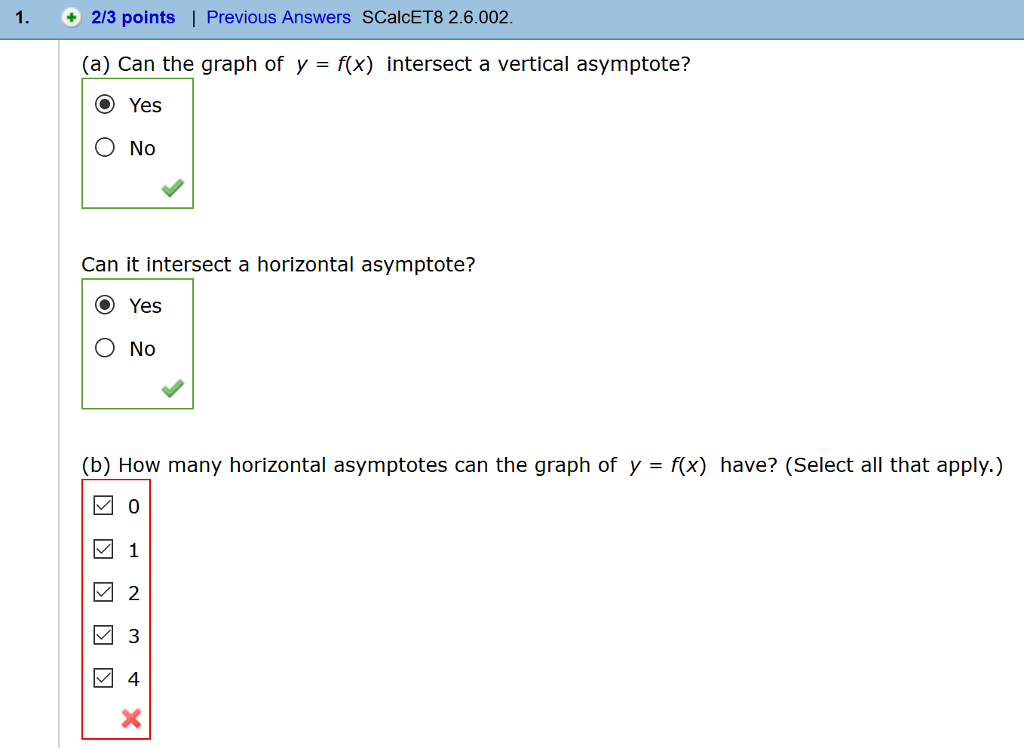
Can a function’s graph intersect a vertical asymptote? To answer this question, we need to consider the concept of continuity. In mathematics, a function is considered continuous at a point ‘a’ in its domain if the limit of the function as ‘x’ approaches ‘a’ is equal to the function’s value at ‘a.’ In other words, lim(x→a) f(x) = f(a).

Now, when it comes to vertical asymptotes, it’s important to understand that these are essentially lines that a function’s graph approaches but never touches or crosses. In order for a function’s graph to intersect a vertical asymptote, it would need to contain a point that lies directly on the asymptote itself. However, due to the definition of continuity mentioned earlier, this is not possible because the limit and the function’s value must match at every point in the domain for the function to be considered continuous. Therefore, a function’s graph cannot intersect a vertical asymptote.