Can A Function Truly Derive Itself? Exploring The Self-Derivative Conundrum
This Function Is Its Own Derivative
Keywords searched by users: Can a function be its own derivative functions whose derivative is itself, why is e to the x its own derivative, a function whose derivative is a constant multiple of itself must be, e^x derivative, function whose third derivative is itself, desmos, find the derivative of sin(ln(x)), what is a derivative in math
Can A Function Be A Derivative Of Itself?
Is it possible for a function to be its own derivative? This intriguing concept is exemplified by the function f(x) = e^x, which holds the unique property of being equal to its own derivative. In mathematical terms, this means that when you calculate the derivative of f(x) = e^x, you obtain the same function, f(x) = e^x, as the result. This phenomenon, where a function’s rate of change is proportional to the function itself, is a defining characteristic of exponential functions like e^x. It’s a fascinating aspect of mathematical analysis and has significant applications in various scientific and engineering fields.
Can A Function Have The Same Derivative?
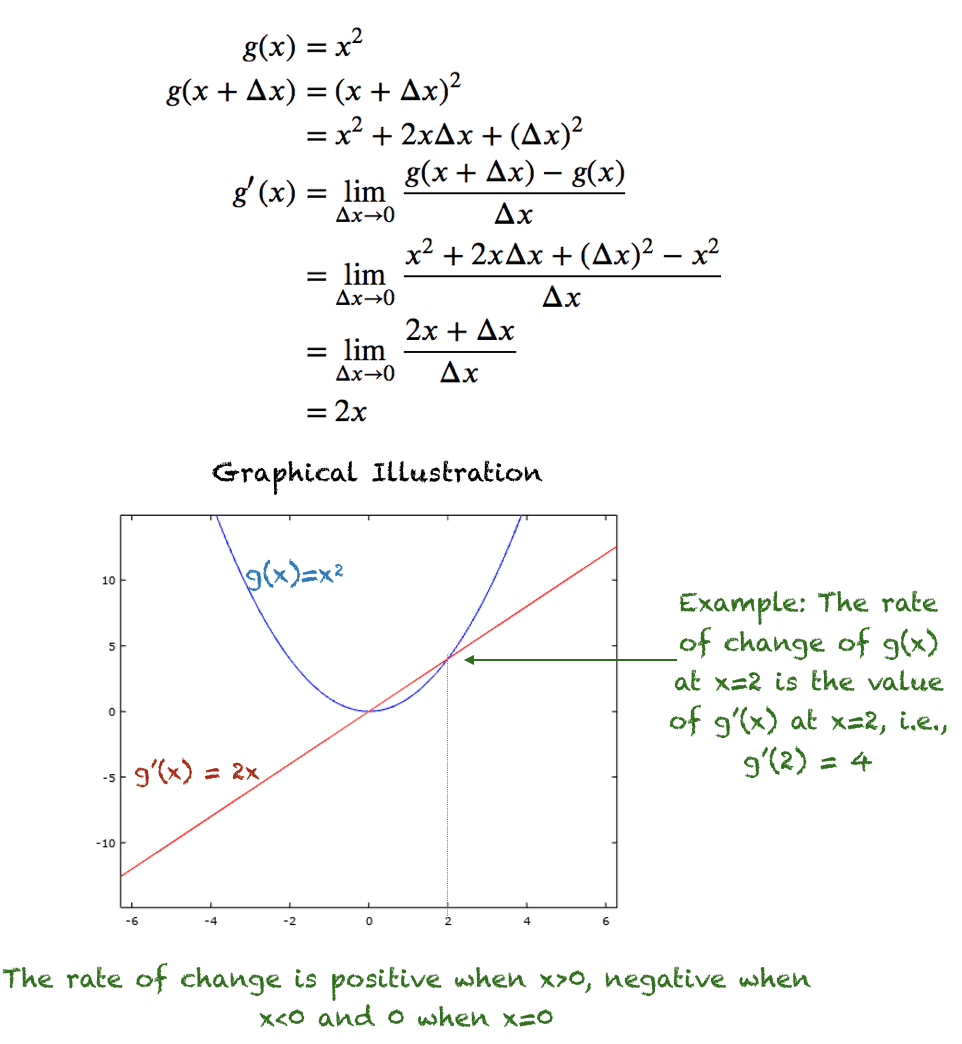
Is it possible for two functions to possess identical derivatives? The Mean Value Theorem (MVT) can shed light on this question and provide a helpful insight. According to the MVT, if two functions share equal derivatives over a particular interval, it implies that these functions are separated by nothing more than a constant difference. In other words, if we have f'(x) = g'(x) for all x within the interval, then the conclusion is that f(x) and g(x) only differ by a constant term. This is an important result derived from the MVT and highlights the relationship between functions with equivalent derivatives and the constant offset between them.
Summary 9 Can a function be its own derivative

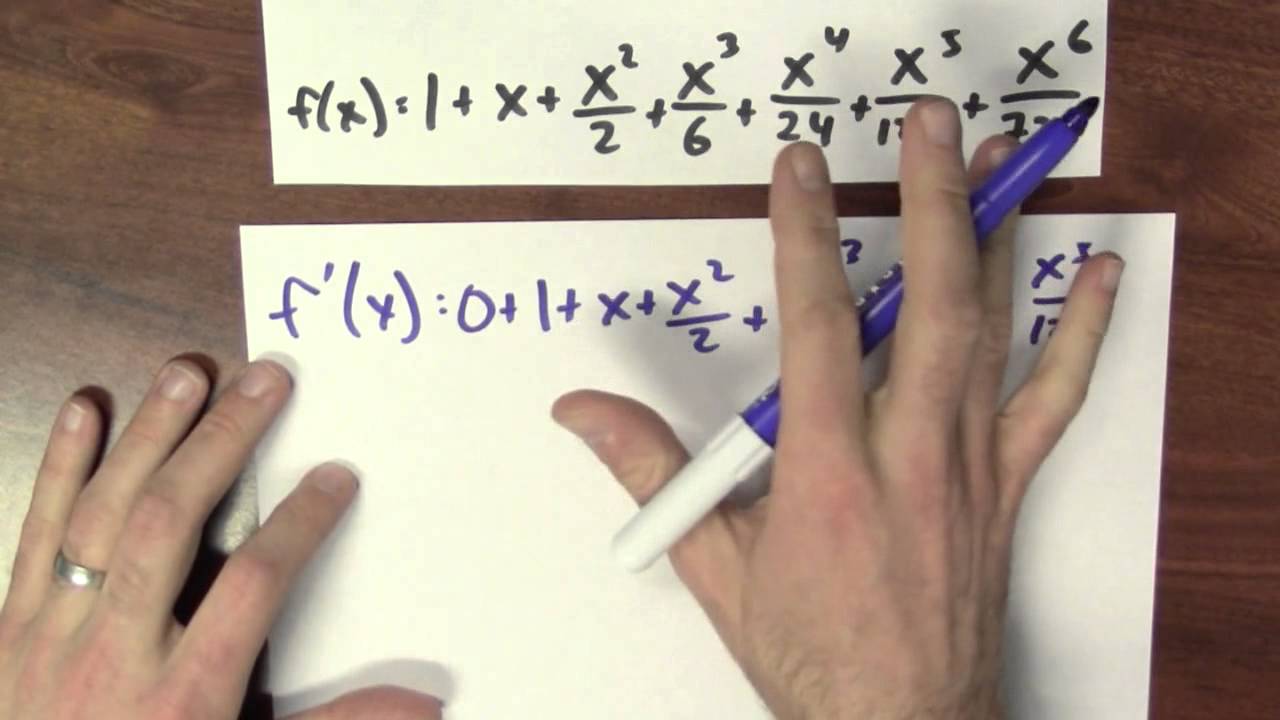





Categories: Summary 79 Can A Function Be Its Own Derivative
See more here: maucongbietthu.com
The derivative of an exponential function is a constant times itself. Using this definition, we see that the function has the following truly remarkable property. Hence is its own derivative.The function f(x)=ex is quite peculiar: it is the only function whose derivative is itself.Functions with equal derivatives. We can also use the MVT to conclude that if two functions have equal derivatives on an interval, then they differ by a constant. For if f/ = g/, then (f – g)/ = 0, therefore, by the preceding theorem, f – g is constant.
Learn more about the topic Can a function be its own derivative.